There is No Gravity in Space
Created March 10, 2014. Post Last Modified August 27, 2022
The Misconception
You’ve seen it in movies. You’ve heard about it somewhere. You probably believe it yourself. You’ve even probably seen live video of astronauts in space floating around! It’s pretty much settled, there must be no gravity in outer space!

To start with, there *is* gravity in outer space. In fact, there is gravity all over the universe. Isaac Newton, and Albert Einstein later on, have clearly established this fact as truth. For this analysis, we are going to focus on Newton’s work.
What the Physics Says
Newton was the first to describe gravitation in detail, and came up with a beautifully short equation to describe how it works.
$F_G=-G\frac{Mm}{r^2}$
where $F_G$ is the gravitational force, $M$ and $m$ are the masses of the two objects being considered, and $r$ is the distance between the centers of mass of the two objects.
This equation can mean many things, so let’s focus on the misconception. If there are no gravitational forces in space, then the left side of the equation must be zero.
$0=-G\frac{Mm}{r^2}$
This implies that at least one of the terms in the right side of the equation must be zero. Let’s check out which one can become zero.
First of all, let’s say that mass of the Earth is $M$. This mass can never be zero, since mass cannot be destroyed (or created). This goes the same for the smaller masses orbiting the Earth, namely the astronaut with mass $m$.

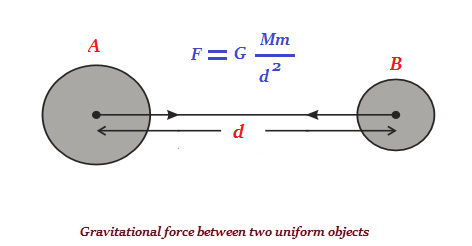

The term $G$ is a constant, with a value of $6.6743 \times 10^{-11} m^3 kg^{−1} s^{−2}$. Since it is a constant, it cannot be zero as well.
This leaves us the term $1/{r^2}$. In most cases, this is the radius of the Earth, since we stay on its surface. An astronaut normally stays between 100 and 200 km above the Earth’s surface. This increases $r$ by this amount, which means that $1/r^2$ becomes smaller. However, we must realize that the value of $1/r^2$ will also never reach zero, no matter how far you get from earth.
Putting everything together, the following equation should be obtained.
$0 = \text{some non-zero value}$
Since we have reached a contradiction, this should mean that the gravitational forces due to the Earth do not completely disappear. Hence the Earth always has a gravitational effect everywhere in the universe, no matter how small! This applies to other masses within the universe as well.
Further Discussion:
Why does it seem like stuff is weightless up there?
How strong is this gravitational pull from the Earth on the orbiting astronaut? The mass of the Earth is $5.97219\times 10^{24}$ kilograms, and its mean radius is $6,371$ km. Using the gravitational force equation and assuming that the mass of an astronaut with equipment is 150 kg, we have the equation
$F_G=-G\frac {Mm}{r^2}$
Since the astronaut is $100$ km above the surface of the Earth, $r = 100+6371 = 6471$ km
$F_G=-6.6743 \times 10^{-11} m^3 kg^{−1} s^{−2}\frac {(5.97219\times 10^{24} kg)(150 kg)}{6,471,000^2 m^2}$
$F_G= 1427 N$
The same astronaut with equipment will experience a gravitational force of $1,473 N$ on the Earth’s surface. Upon comparison, the force in space may not be strong as on the surface, but it’s definitely close! So why does it seem like there is no gravity up there? To answer this, we’ll do a thought experiment similar to what Einstein himself used to come up with the general theory of relativity.


Imagine that you are floating around in a large truck container with no
windows or openings to the outside, and you do not know where in the
universe you are. Just by what you can see inside the container, can
you determine whether you are floating in zero gravity or falling to the ground?
(Einstein used the opposite effect, that is, if you were in the
container and are standing up in it, can you determine whether you are
on the Earth’s surface or accelerating upward?)
Most people would say, “I wouldn’t be able to know”. Just like these people.
The people in the airplane experience what seems to be zero gravity, but they are actually falling due to gravity. It’s just that the airplane (the “container”) is falling with them. This removes any contact with the floor, and with it the removal of any contact forces that will counteract your weight. The contact forces are what make you feel your weight. Without any contact forces, you feel weightless.
A smaller effect happens when you are in an elevator. When it starts to go down, you feel lighter, since the floor does not support you as much as before moving. When you slow down and stop, you feel heavier because the contact forces on you are larger.
It’s a similar thing that happens with astronauts, but with one key difference: They are orbiting the earth instead of falling to the ground.
Orbiting may seem very different from falling, but it’s actually the same thing, with an additional motion perpendicular to falling (i.e. moving forward and falling downward at the same time). Imagine that the Earth suddenly disappears. The astronauts (and everything else) orbiting the Earth will stop moving in circular (or more accurately, elliptical) orbits and move in a straight line. The combination of falling towards the Earth due to gravity and the perpendicular motion makes the astronauts move in an approximately circular orbit around the Earth.
In either case, the overall effect is the same. The lack of contact with the floor makes you (any anything, really) feel like you are weightless.
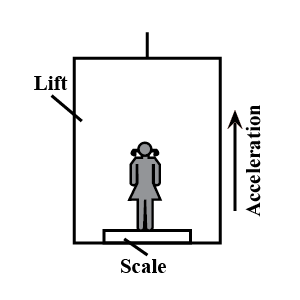
Summary
Being in space means that there is gravity acting everywhere, just like on Earth. The sensation of weightlessness you would experience is actually not because of the lack of gravity, but because the sensation caused by moving with your container makes you think that are floating around. The fact is that you are falling, but can’t sense it because of the lack of contact forces with the floor.
Anything still not clear? Leave a comment below!
Sources
- https://www.aps.org/publications/apsnews/200507/erastosthenes.cfm
- https://en.wikipedia.org/wiki/Earth_mass
- https://www.esa.int/Science_Exploration/Human_and_Robotic_Exploration/Astronauts/Granting_weightless_wishes
- https://www.youtube.com/watch?v=SAQ-iIJkLzA
- https://www.youtube.com/watch?v=LWGJA9i18Co
- https://www.toppr.com/ask/question/mandy-stands-on-a-weighing-scale-inside-a-lift-elevator-that-accelerates-vertically-upwards-as/
